Solving Word Problems…a Gradual Release Model

For many students, word problems and problem-solving strategies are the most difficult part of math instruction. They can learn algorithms and procedures but struggle to independently make sense of problems and work through them. So what can we, as teachers, do to help? Let me walk you my thinking and see if there is anything that resonates with you!

Gradual Release of Responsibility Model for Word Problems

For those of you who are unfamiliar with the work of Fisher and Frey, I strongly encourage you to dig in. The research is sound, and the methods are practical. In a nutshell, the GRR involves a shifting of “cognitive work” from the teacher to the student. It is not a linear process (a common misconception), but a cyclical, ongoing practice where the teacher provides explicit teaching first. After this, opportunities for questioning and prompting of students as they work in small groups help guide students toward deeper understanding. Teachers can intervene as needed, return to the targeting lesson content, and then back away. Finally, we want students to be able to do the work independently and apply their understanding to novel situations. This is where problem solving and the standard “Makes sense of problems” comes into play. It’s also where things often break down.

The Importance of Modeling our Think Alouds

When we hear “modeling” in math, we often think of modeling with objects. This, of course, is exceptionally valuable. It may be super relevant with word problems as well if students need concrete objects to model the actions of the problem. Where I think we sometimes drop the ball is with respect to modeling our thinking. I think we DO this…but I think we sometimes don’t model the right thoughts. Check out the difference between these two think alouds. They aren’t dramatically different–but the second one does much more coaching about the “WHY”, not just the HOW. See if you can tell what I mean.

“So first I added the $4.55 to $2.95 twice to get the total amount she spent on candy.” (Tells HOW the teacher solved the problem.)

“I knew that there were three bags of candy that she wanted to buy. One cost $4.55. The other two bags cost $2.95 each. I know I need to add that $2.95 two times to make sure I get all three bags of candy included in my total.” (Tells WHY the teacher solved the problem and HOW his/her thoughts were organized.)

The Importance of Students Sharing Their Thinking

So, in addition to us being truly mindful of how we share our thinking–which is, in essence, a form of coaching, we must also provide ways for students to share their thinking. This is important for so many reasons.

Students deepen their understanding when they talk about their work.
Students can help others by explaining strategies in a new way.
Misconceptions can be uncovered and redirected.
The classroom is full of teachers. The more people talking, the more learning is occurring.
Research suggests that math class should be at least 60% discourse among students.
It is far more efficient for students to be a part of a learning community where they work collaboratively than to have to wait for a teacher’s guidance.
Students are more likely to be engaged when they are active participants.

Because of this, it is absolutely essential that we get students talking about math. We need to model how to do it–then provide opportunities for practice.

Large group sharing

There are tons of ways for students to share in a large group setting. Students can put work samples under a document camera and explain their thinking. Students can simply raise their hands and share strategies. When we do number talks, we can get all sorts of student thinking exposed to the entire class. We can even do things like gallery walks where students can see work samples from lots of students. There are pros and cons to any large group work, but this is certainly an important part of giving students practice sharing their ideas and listening to and learning from others.

Small group sharing

Sometimes we can get more students sharing–and more students listening–by using small groups. Whether you help coach students on how to work in their desk groups or if you have students do a lot of work in partners, we can give explicit coaching on how to take turns, share ideas, ask for clarification, agree and disagree, and much more. Providing displayed sentence starters for this “accountable talk” is super helpful. By making this a part of every day’s math instruction, students learn to expect it and get better and better.

We, as teachers, can circulate as spies. We listen for things to reinforce (“I love how Becca told WHY she multiplied because that makes sense to me.”) as well as intervening when misconceptions occur (“So is it possible to take 46 doughnuts and put them into 4 equal groups? Can you prove that?”). So often teachers forget how important our presence is during these small group sharing times.

One-on-one sharing

Sometimes it’s important to work one on one with a student to see exactly what they know and where understanding might be breaking down. Listening to students “think aloud” is a great way to intervene right at the point where meaning breaks down and to better see what tools students actually DO have in their toolbox. Sometimes we wait too long to diagnosis when a quick one-on-one conference can shed some light into student thinking.

How I Teach Problem Solving with Word Problems

There are tons of problem solving acronyms out there that are supposed to make it easier for students to solve problems. I have some concerns about acronyms (ex “CUBES”) that turn into cute anchor charts but aren’t explicitly taught. I’ve often felt like many of these problem solving “strategies” don’t really do much in terms of really helping students make sense of problems. They are short and to the point–and that has its merits, of course. That being said, I want a tool that actually helps me teach students to be better problem solvers. I don’t want my students to say, “Oh, I used CUBES to solve the problem.” I want them to be able to do SO MUCH problem solving that these steps because automatic. Until that point, I need to teach and model and coach them through the steps.

It takes YEARS for students to become masterful problem solvers–and as they move up in years at school, the math content of the problems becomes more complex and these steps become all the more important. So here’s what I do. Like with the gradual release model, students need to understand that these steps aren’t necessarily linear; they are “recursive”. This means they may need to revisit previous steps at different points–and that’s what good problem solvers do.

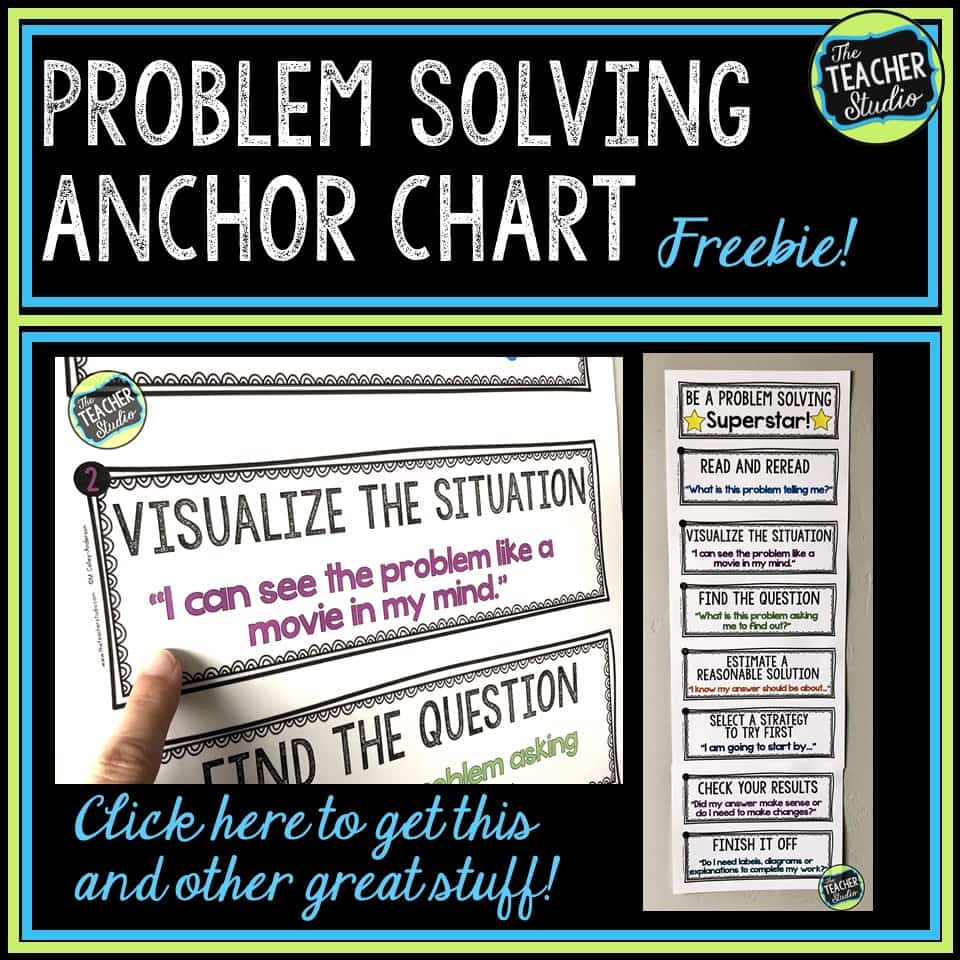
Read and reread
Visualize the situation
Find the question
Estimate a reasonable solution
Select a strategy to try first
Check your results
Finish it off

It doesn’t make a very good acronym, does it? It also isn’t super helpful for students–unless each of these steps is taught and retaught and modeled over and over. So I like to turn these steps into student-friendly language as a part of the process. See what you think about this version–and my explanations to you about the steps and why they are so important.

Read and reread

becomes “What is this problem telling me?” We want students to know that reading a math problem requires them to slow down and read carefully–and perhaps multiple times. They need to be on a quest to discover what information the problem is telling them–and possibly find information that isn’t even relevant.

Visualize the situation

becomes “I can see the problem like a movie in my mind.” Visualizing is a strategy frequently taught and practiced during reading instruction but is often overlooked in math. When a problem says, “Michael put three pans of cookies in the oven. Each pan has 12 cookies.”, students need to be able to “see” that so they know that we are talking about multiple groups of 12. If the problem stated, “Michael had 12 cookies. He put them into three boxes.”, we want students to “see” him sharing the cookies out into groups as a division problem.

So many math errors happen because students fail to choose the correct operation. We teach students incorrectly to look for signal words like “total” instead of showing them how to visualize what is happening and to choose an operation to match. This is also essential for helping students to know if their answer is reasonable. Sometimes I close my eyes after reading a problem and say things like, “I see a bowl of 100 jelly beans. Try to imagine me taking out three handfuls of 20. I know the amount in the bowl is getting smaller so my answer needs to be less than 100.” You get my point.

Find the question

becomes “What is the problem asking me to find out?” So often students fail to understand that many problems COULD have lots of questions asked about them. Consider the following situation:

Marcus bikes to school and home again every day. The trip is exactly 1.5 miles. It takes him 10 minutes to bike each way.

By presenting a problem such as this WITHOUT a question, we can ask students to generate a list of questions that COULD be asked with the information presented. For example, this problem could generate questions such as…

How many miles does Marcus bike each week?
How many minutes does he spend biking each week?
If school starts at 8:00, what time should he leave his house?
How many miles does he bike each day?
If his friend lives 0.4 miles closer to school, how much farther does Marcus bike than his friend each day?

You get my point. The more we expose students to problems like this, they start to get creative with thinking about questions–and as a result, they become far more “clued in” to the actual questions being asked in problems they encounter. I love hearing students say things like, “I was thinking the answer was ___ , but I knew that wasn’t the question is was asking.” #forthewin

Estimate a reasonable solution

becomes “I know my answer should be about…” I think this is pretty self-explanatory. We want students to have an IDEA of what an answer should be. Is the answer supposed to be bigger? Smaller? Should it be bigger than my starting number? Is it possible to put 4,288 cookies in the oven at once? Does it make sense to order 634 pizzas for a party? By helping model our thinking, students become better at catching errors that simply don’t make sense.

Select a strategy to try first

becomes “I am going to start by…” We want students to have a bunch of tools in their toolbox. They can start by drawing a picture. Grabbing manipulatives. Choosing an operation to try. There are tons of strategies to use. (By the way, CLICK HERE for a blog post with more ideas about this.) Students need to have enough ideas to get started–and then to readjust if their first strategy didn’t work. We can model this with our gradual release. “I started to draw a picture of this problem and realized that the numbers were too big. I think I’m going to try repeated adding to see if that works. Does anyone have any other starting strategies for me?”

Check your results

becomes “Did my answer make sense or do I need to make changes?” You know elementary students. Once they have written a paragraph, read a book, or solved a problem, they are FINISHED. We need to coach them on going back to study their work to see if they really are done, if their answer is reasonable, or if they need to try a different approach. Using think alouds as a teacher is key. “I doubled checked the problem and my answer seems reasonable. I know that if I had a pan of 24 brownies and I gave 1/3 away, that I will have less than 24 left. My drawing shows the 24 brownies split into three groups and my labels show which brownies are mine, and which are given away. I think I’m correct.”

Finish it off

becomes “Do I need labels, diagrams, or explanations to make my work complete?” This final step is one my students struggle with–but I think is key. It has to do with precision and making work “professional”. I want students to have their work organized–even if the process is messy along the way when they finish their answer should be clear as a bell. I talk to students about ways to showcase their answer, perhaps by circling or boxing it. Sometimes they may need to skip a line and rewrite it. I also want them to get used to adding labels and drawings to help, and–when needed–explanations of what they did. Before they say, “I’m DONE!”, I want them to make sure they are proud of what they did and that others can understand exactly what they did.

Problem Solving Anchor Chart

One thing to consider is to have a display…maybe an anchor chart or series of pictures to help students remember all these key steps. This could be something super informal or something preprinted like the image below. I would also recommend that each step of this process be explicitly taught, modeled, and practiced–using that Gradual Release method. Finally, consider giving students a handout to keep as a reference–something they can refer to when they need support. Shhhh…I may have something that can help! See below.

A Problem Solving Freebie for You!

So I thought I’d put these strategies into a little freebie for you in case you wanted to try something new with your students! Feel free to print off these problem solving “steps” and put them together like an anchor chart to be used as a reference tool. There is also a one-page student sheet that can be given to students as a tool to help them when working through this process. See what you think by clicking below!

Solving Word Problems…a Gradual Release Model

Gradual Release of Responsibility Model for Word Problems

The Importance of Modeling our Think Alouds