The first part of our next unit in math involves helping students derive the area and perimeter formulas for rectangles. Knowing what I know after doing this little “gig” for 20 something years, I was pretty confident that I needed to find out if, indeed, my students REALLY knew what a rectangle was. Simple right?
False.
I thought I’d start with asking students to jot down their thoughts about the following two questions:
What do you know about rectangles?
What do you know about measuring rectangles?
As I walked around, I grew increasingly glad that I didn’t jump right into the first lesson of this unit! We had some foundations to build!
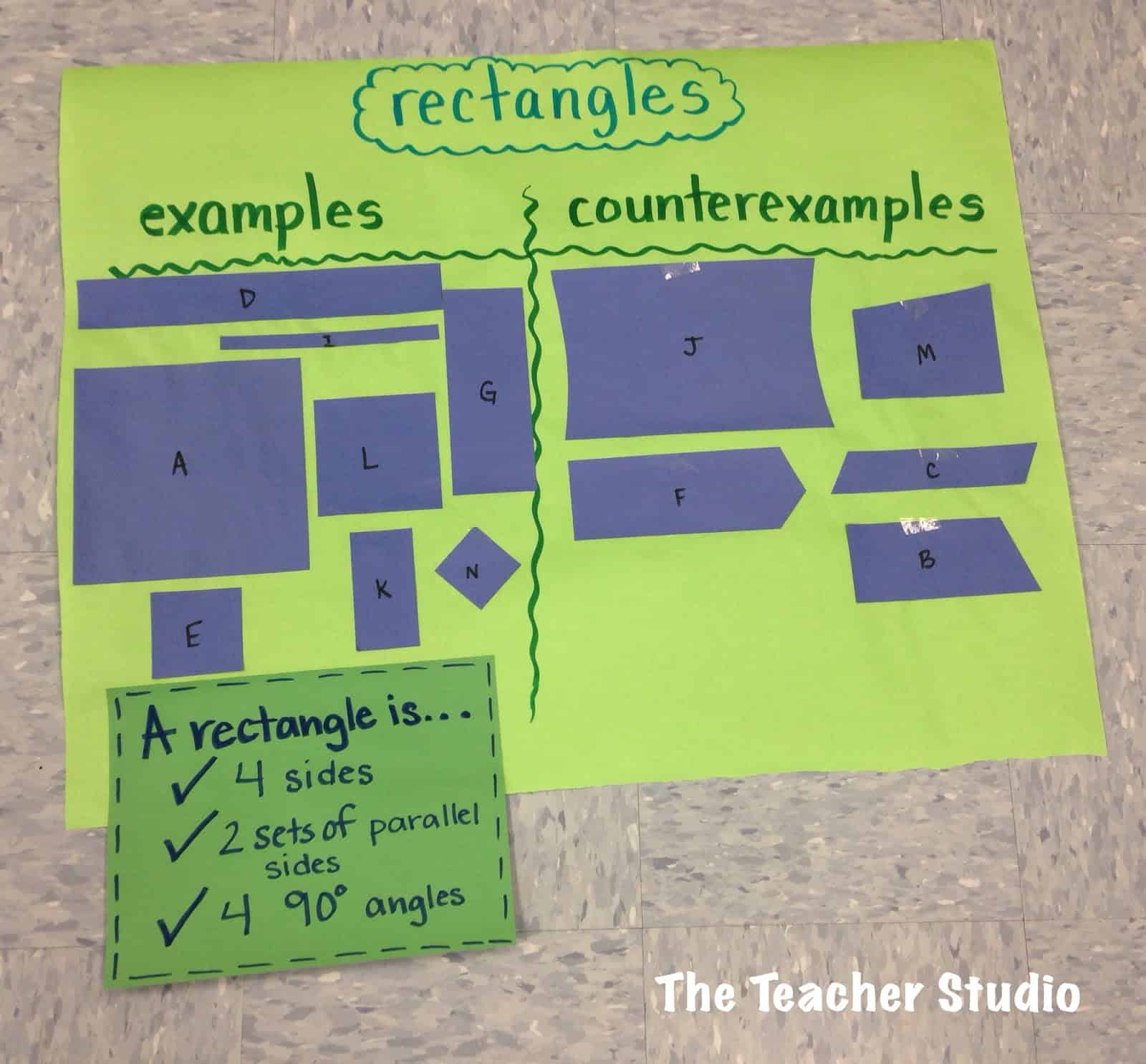
I brought us back together as a class and began showing the students some shapes that I had cut out of construction paper. One at a time, I held them up and we “played democracy”–majority ruled. The question? Is it a rectangle–or is it a “counterexample”. Here’s what we ended up with.
Notice anything? Yep. MAJOR misconception! My students did what I expected…they identified the “classic” rectangle by visual shape without really understanding what characteristics a rectangle must have. So…here begins the discussion.
First, I asked students to help me select which of the shapes they were 100% confident were NOT rectangles…and to explain why. We worked our way through the counterexamples and found out that shapes with curved sides can’t be rectangles…shapes with 5 sides can’t be rectangles…and eventually agreed that shapes without right angles couldn’t be rectangles. (Some were very upset by this and were pretty sure that “C” was a rectangle…they remembered that the opposite sides should be equal and that letter C fit the rule. I intervened with what was becoming a hot debate and reinforced that rectangles do, indeed, need four right angles.) Here’s what we ended up with.
 |
| Sorry so blurry! Didn’t realize it at the time…and didn’t take a second shot! |
Interesting, right? Time to come up with our set of rules. Can a square be a rectangle? Can a “diamond” (pet peeve…I had to do my “If I turn Megan upside down or on her side, is she still Megan?” talk.) Students began talking and generating rules and ideas and after a few minutes I wrote them down. Now…if you are a geometry purist, don’t be alarmed. I know there are more precise definitions out there–but this is what my students decided on, and they do work to help us define rectangles!
1. It must have 4 sides.
2. It must have 4 right angles.
3. It must have two sets of opposite parallel sides.
We had QUITE the discussion about squares today and finally determined that a square IS indeed a rectangle-but that it is a SNOBBY rectangle that thinks it’s better than other rectangles because all four sides are perfectly equal. Will they remember that a square is a snobby rectangle? I hope so!
We worked through the shapes then and reorganized to find the TRUE examples of rectangles.
So…next steps? I asked the students to process the information they had learned in their notebooks. I asked them first to write about what they know to be true about rectangles, and then to make their own rectangle/not rectangle T-chart. We’ll see what they remember in a few days!
Want some more help looking for misconceptions in the area of geometry? Try working with concept sorts…and here is a set of 5 geometry sorts that give you some great guidance to get started!
Next up? Digging into area and perimeter. Stay tuned!