We are moving into our mini-unit on factors, multiples, prime numbers, and factoring–SO much vocabulary and such complicated ideas for students who may or may not be secure with their multiplication facts. To really try to help students “SEE” the concepts, I believe super strongly in using concrete models.
Seriously though…I really want my students to deeply understand the concept of arrays and how they relate to multiplication, factors, products–and eventually prime and composite numbers.
So the other day I asked my students to tell me what they knew about arrays–and they shared out their ideas. They DID have a bit of a hard time using precise mathematical language so I flipped on the projector and document camera, whipped this Dove monster out of my school bag, dramatically wafted the smell toward them, and threw it under the camera asking, “Will THIS help you describe what an array is?” After a few moments of whining about how it wasn’t fair that I had one and they didn’t, we got to work using clear math language to describe this array. We used the words factor and product and array. We talked about directionality (Is 6 rows of 3 the same as 3 rows of 6? Is 6 bags of 3 apples the same as 6 bags of 3 apples?)
I then pulled out a bag of these to a roar of delight from the crowd and asked to practice that mathematical language to describe THIS array. The results were much more clear!
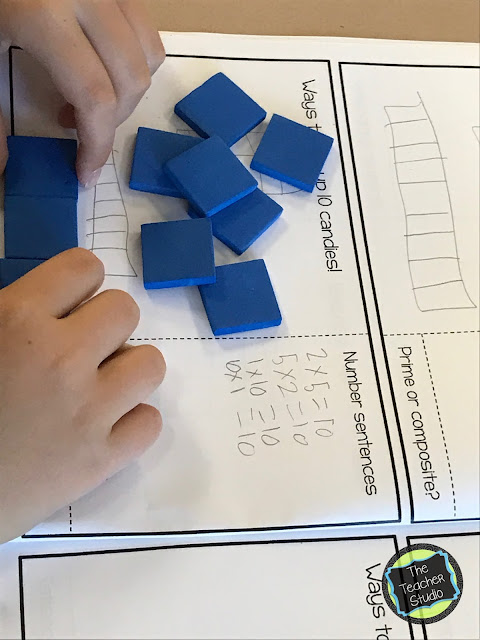
After consuming a little “Brain Food”, we got to work on one of my favorite hands-on lessons of the year–the Candy Factory! In this project, we arrange candy “tiles” in all the possible arrays trying to look for patterns…we uncover the “double/half” strategy, started to notice some thing about arrays with odd numbers, and more!
As students moved forward from making “boxes” of one candy…then two candies…then three, you could feel their confidence growing–and their willingness to model with the tiles diminishing. I continued to encourage them to use them…but some were insistent that they could do it in their head. I was noticing everyone getting the “one by…” arrays (1 x 12… 1 x 13…and so on) but were starting to miss some of the “short, chubby” rectangles. Some students had even made a claim that “All odd products only have 2 factors.” Hmmmmmm
But it was when I came upon this example that I knew we had problems.
Big. Problems.
So at this point, I INSISTED that he break out the tiles and SHOW me the array he had drawn. As you can imagine, he wasn’t able to do so and a little light bulb went off. I asked him how he thought he COULD arrange the 9 tiles and, after some experimenting, figured out that, in addition to the 1 x 9 array, he could also build a 3 x 3. He could NOT do it in his head…he needed that tangible tool–FOR NOW. I asked him if I could share his story with the class so we could all learn from it and he agreed.